Prime Or Composite: Understanding the Fundamentals of Number Classification

Number classification is one of the most important concepts in mathematics. It helps to distinguish the natural numbers from the integers.

 

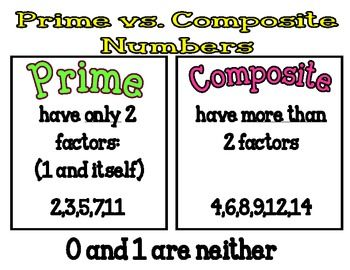
A number is classified as a prime or composite number depending on the factors it has. Prime numbers have only two factors whereas composite numbers have more than two factors.

Definition of prime and composite numbers

Prime or Composite: Understanding the Fundamentals of Number Classification

To identify a number as a prime or a composite, you need to know a few basic properties. These include how many factors a number has, how rare a number is, and whether or not it is even or odd.

 

A prime number has exactly two factors - 1 and the number itself. This means that the number can be divided only by 1 and itself.

 

Unlike prime numbers, composite numbers have more than two factors. They are those integers that can be generated by multiplying the two smallest positive numbers and include at least one divisor other than 1.

 

This is why mathematicians use composite numbers to express various patterns and number concepts. In addition, computers use composite numbers and factorization to create encryption algorithms that protect confidential information.

If a number is divisible by 2, 3, 5, 7, 11, and 13, it’s prime; otherwise, it’s composite. This is because 2 is the only even prime number.

 

However, there are a few odd composite numbers. You can see them highlighted in blue in the chart below.

The number 185 is not a prime because it ends in 5. It’s also not an even number, so it’s composite. This is why you should always look for even and odd digits when determining whether or not a number is prime or composite.

Basic properties of composite numbers

If a positive number is divisible by more than one positive integer, it is classified as a composite number. These numbers are considered the opposite of prime numbers.

 

The basic properties of composite numbers are very simple and easy to remember. They are also used in many areas of mathematics and science, including graph theory and cryptography.

 

Generally, all even numbers are composites because they are divisible by more than two numbers. This is why we can say that the smallest composite number is 4.

 

It has three factors: 1, 2, and 4 itself. The number 6 is another natural number that has more than two factors, which means it is a composite number.

 

So, if you want to know whether a number is a composite number or not, first find out all the factors of that number. The factors of 16 are 1., 2., 4, 8, and 16.

 

Similarly, the factors of 34 and 39 are 3, 13, and 38. The factors of 43 are 3, 6, and 13. All the numbers that have more than two factors are composites.

Factorization of composite numbers

A number that is divisible by more than two numbers other than 1 and itself is called a composite number. These are all the natural numbers that have more than two factors (such as 6, whose factors are 1, 2, 3, and 6).

 

The only number that isn't a composite number is 1; it only has one factor. However, if the only factors a number are 1 and itself, that is a prime number.

 

To determine whether a number is a composite or not, perform the divisibility test. For example, 48 is divisible by 2 and 3.

Another way to find out if a number is a composite is by finding the smallest composite number. If it is, then we can use the trial division method to factorize it.

 

In this method, you start by dividing the number by the smallest prime. Then you repeat the process until the quotient no longer divides evenly. You will end up with a list of prime factors.

 

Students can practice this by using the beginning number cards that have each number 1-100 listed as a prime or a composite. They can also learn the factorization of a composite number on their own.

 

Prime numbers have an important place in mathematics. They are the building blocks of all integers, and they have fascinating applications in physics, music, and even cryptography. They are especially difficult to factorize, which makes them a key component of computer security.

Common factors and greatest common divisor

A common factor is the largest number that divides evenly into two other numbers. It is also called greatest common divisor (GCD). In arithmetic, the GCD of two numbers can be calculated by decomposing them into their factors and then comparing them.

 

The greatest common divisor of two or more numbers a and b is a positive integer m, which divides both a and b. The GCD is commonly written gcd(a, b).

 

In ring theory, the concept of a greatest common divisor can be generalized to a principal ideal generated by (a, b). For rings for which there is an analogue of the Euclidean algorithm, the gcd of a and b is often known as the preorder relation of divisibility.

 

There are some exceptions to this rule, such as coprime numbers. However, these are rare and may be hard to find.

Another important example is the ring of polynomials. The gcd of two polynomials is unique up to a certain factor, and it is a principal ideal.

 

The GCD of two numbers a and b is the highest positive integer m that divides both a and b. This can be computed using prime factorization or the Euclidean algorithm.

Cryptography and prime numbers

Prime numbers are a very important part of cryptography. For instance, a common encryption method involves using the product of two large primes to derive a public key (for encryption) and a private key (for decryption). This makes it difficult for unwanted people to read your encrypted information.

 

There are many reasons why prime numbers are so important in cryptography. For one, primes have special properties that make it very difficult to factor a large number into a number of its factors. For example, it's not easy to find a prime factor for 20; it's impossible to factor 2,244,354.

 

Another reason is that primes are easily tested to see if they are prime, which makes them ideal tools for encryption. There are also more advanced primality tests that are faster than traditional methods for checking a prime number, so if you need to send an encrypted message, it's best to use a good primality checker.

 

Primes are also critical to RSA, the most popular type of encryption used today. The RSA algorithm uses the product of two large primes as its encryption and decryption keys, making it very difficult to break.

Conclusion

Numbers are classified as prime or composite according to the factors they have. The first 5 numbers are called primes as they have only 2 factors, 1 and the number itself. The other numbers are known as composites as they have more than 2 factors.

 

There are also co-primes which can be defined as a number that has exactly one number as a common factor. These numbers can be used in many math applications.

 

To get a clear picture of these two types of numbers, it is necessary to understand the fundamentals of number classification. The most important of these is Prime or Composite.

 

The first and foremost distinction is that a prime number has exactly 2 factors. It cannot be divided by more than these.

However, composite numbers have more than 2 factors and can be divided by at least one positive number. They are further categorized as odd and even composite numbers.

 

Another important point to remember is that all odd numbers are not prime numbers and all even numbers are divisible by 2. Hence, we can conclude that a number less than 289 is a prime whereas all other numbers can be classified as composite.

 

It is therefore essential to know which numbers are prime and which ones are not. This can be done by using the prime factorization method and divisibility test. There are also a few other ways of determining whether a number is a prime or composite, but these techniques are often not as accurate.

FAQ’s

Q: What is a prime number?

A prime number is a positive integer greater than 1 that is only divisible by 1 and itself. Examples of prime numbers include 2, 3, 5, 7, 11, and 13.

 

Q: What is a composite number?

A composite number is a positive integer greater than 1 that is not a prime number, meaning it has at least one other factor besides 1 and itself. Examples of composite numbers include 4, 6, 8, 9, 10, and 12.